Vaccination-based Measles Outbreak Model with Fractional Dynamics

Authors

  • Mohammed S. Abdo Department of Mathematics, Faculty of Education, Hodeidah University, Yemen
  • Mohammed Amood AL Kamarany Department of Pharmacy Practice , Faculty of Clinical Pharmacy – Hodeidah University , Hodeidah , Yemen and Center of Tropical Medicine and Epidemiology Studies, Hodeidah University (CTMES - HU), Hodeidah , Yemen
  • Khaled Ahmed Suhail Center of Tropical Medicine and Infectious Diseases , Authority of Public AL Thawarah Hospital , Hodeidah , Yemen
  • Ahmed Suliman Majam

DOI:

https://doi.org/10.59846/ajbas.v1i2.439

Keywords:

Measles, Outbreak, Mathematical Model, Fractional Dynamic, fixed point theorem

Abstract

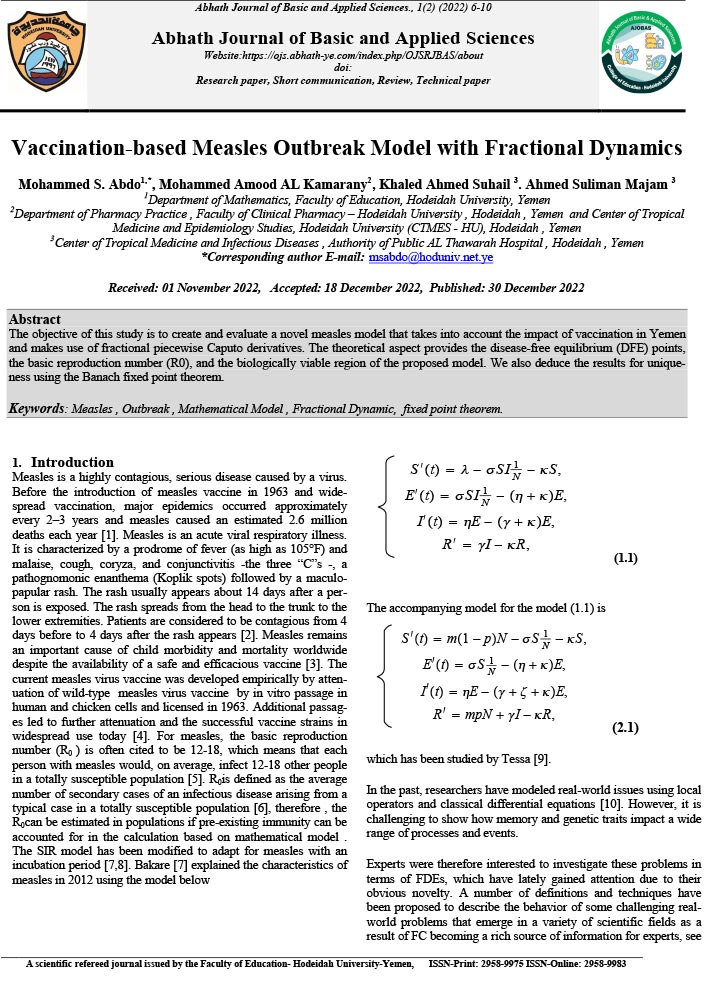
The objective of this study is to create and evaluate a novel measles model that takes into account the impact of vaccination in Yemen and makes use of fractional piecewise Caputo derivatives. The theoretical aspect provides the disease-free equilibrium (DFE) points, the basic reproduction number (R0), and the biologically viable region of the proposed model. We also deduce the results for uniqueness using the Banach fixed point theorem

Vaccination-based Measles Outbreak Model with Fractional Dynamics

Downloads

Published

30-12-2022

How to Cite

Mohammed S. Abdo, Mohammed Amood AL Kamarany, Khaled Ahmed Suhail, & Ahmed Suliman Majam. (2022). Vaccination-based Measles Outbreak Model with Fractional Dynamics. Abhath Journal of Basic and Applied Sciences, 1(2), 6–10. https://doi.org/10.59846/ajbas.v1i2.439

Issue

Vol. 1 No. 2 (2022): Volume 1 - Issue 2

Section

Articles

License

Copyright (c) 2022 Mohammed S. Abdo, Mohammed Amood AL Kamarany, Khaled Ahmed Suhail, Ahmed Suliman Majam

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.